Lloyd Rieber's home page

1998 Peter Dean Lecture

What is really true? An Exercise in Understanding Constructivism

When discussing philosophical orientations and their implications to learning and education, I like to use this little exercise. It begins with two questions: 1) consider and declare what was your favorite subject when you were a student in public school (any grade through high school); 2) pick one thing from that subject you believe to be irrefutable, that is, something that everyone (even an expert) agrees to be true. This leads to all sorts of interesting statements of fact, ranging from mathematics (e.g. 2+2=4) to language (e.g. each sentence must contain a subject and a verb) and history (e.g. Columbus discovered America in 1492). However, there is one final question that I then ask: Explain how you, personally, know it to be true. Put another way, how would you convince a skeptic of its truthfulness or validity? This is a rather unnerving challenge. So many things we accept to be true without really questioning the validity of the statements. Good examples come from math and science, such as the world is round and it travels around the sun. I read somewhere that someone once asked the philosopher Ludwig Wittgenstein how it could have been people could have been so stupid to have believed that the sun revolved around the earth. He is said to have responded, "I agree, but I wonder what it would look like if the sun really did revolve around the earth?" The point being, that it would look exactly the same.

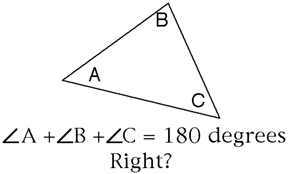Here's one fact that I dare say would be judged by most people as being true beyond refute: "The sum of the interior angles of any triangle equals 180 degrees." This is something we all learned in school. But how do you know it to be true? You could draw a triangle on a sheet of paper and measure the angles. But this is just one triangle, how do you know it to be true of all triangles? So, you draw many triangles, measuring each one carefully. Perhaps you even use a tool such as Geometer's Sketchpad which allows you to construct and test literally thousands of triangles in a matter of seconds. But those are just small triangles drawn on paper or the computer screen,. How do you know it to be also true of larger triangles? Perhaps you take some string and construct triangles that fill a table, a room, or someone's backyard. But these are still relatively small triangles, what about really big triangles, measuring miles along a side? Let's jump to an extreme case. If you start at the north pole and stretch a piece of string to the equator, turn left 90 degrees, walk around one quarter of the way around the globe, then turn left 90 degrees again you will eventually find yourself back at the north pole. The triangle you have constructed will have three interior angles measuring 270 degrees! Of course, you will say that I have tricked you and that the curvature of the earth got in the way. That argument only makes sense when you have a certain perspective on things, because as you are pulling the string, it certainly appears straight from where you are standing. A new perspective or understanding can lead to a changing of the "truth." (Incidentally, this is not really a trick. Einstein's General Theory of Relativity, as I understand it, says that space is curved in the presence of a gravitational field, so the angles of triangles, even as you originally understand them, do not *really* measure 180 degrees.) It is at this point in the class that I like to bring up Von Glaserfeld's concept of "viability versus truth," because many ideas are viable in the everyday world and ought to be taught. Another good example is Newton's laws of motion. These are still viable, even though they are no longer considered "true" by physicists, because they have practical uses.

So, as our understanding of something increases, truth itself can change. The phrase "You are what you know" aptly captures the importance of epistemological questions, such as what does it mean to "know" something. However, curricular questions about what should be taught and why are not always perfectly aligned with the "truthfulness" of the content.

Click here to free of charge from Macromedia.

Copyright 1998 Lloyd Rieber

Lloyd Rieber's home page

1998 Peter Dean Lecture